Gamma

How Delta is expected to change given a $1 move in the underlying is called Gamma. An investor can see how the Delta will affect an option's price given a $1 move in the underlying, but to see how the Delta on that option might change given the same $1.00 move, we refer to Gamma. Gamma will be a number anywhere from 0 to 1.00. Since Delta cannot be over 1.00, Gamma cannot be greater than 1.00 either as Gamma represents the anticipated change in Delta.

Looking at a hypothetical example, XYZ is trading 50. The XYZ Jan 50 call is trading for $2, has a Delta of .50 and a Gamma of .06. Should XYZ go up to $51, an investor can estimate that the 50 strike call will now be worth around $2.50. The new Delta of this 50 strike call at an XYZ price of $51 should be around 0.56 (simply adding the Gamma of .06 to the old Delta of .50).

In other examples

For the purpose of adjusting Delta amounts, round Gamma to two decimal places

• A call has a Delta of .54 and Gamma of .0400 (.04)
  • Stock goes up $1
  • Delta will become more positive by the Gamma amount
  • New Delta value: .58
• Another call has a Delta of .75 and Gamma of .0340 (.03)
  • Stock is down $1
  • Delta will become less positive by Gamma amount
  • New approximate Delta value: .72

Long options, either calls or puts, always yield positive Gamma. Short calls and short puts will have negative Gamma. Underlying stock positions will not have Gamma because their Delta is always 1.00 (long) or -1.00 (short) and will not change. Positive Gamma means that the Delta of long calls will become more positive and move toward +1.00 when the stock price rises, and less positive and move toward 0 when the stock price falls. Long Gamma also means that the Delta of a long put will become more negative and move toward –1.00 if the stock price falls, and less negative and move toward 0 when the stock price rises. For a short call with negative Gamma, the Delta will become more negative as the stock rises, and less negative as it drops.

Gamma is higher for options that are at-the-money and closer to expiration. A front-month, at-the-money option will have more Gamma than a LEAPS® option with the same strike because the Delta of the near term options move toward either 0 or 1.00 is imminent. With higher Gamma, investors can see more dramatic shifts in Delta as the underlying moves, especially with the underlying around the strike at expiration.

Gamma is lower in the longer-dated LEAPS® as more strikes remain possibilities for being in-the-money at expiration because of the amount of time remaining. An at-the-money-option’s Delta is typically the most sensitive to moves in the underlying (hence higher Gamma). With the stock right at a strike at expiration, an option’s Gamma will be at its highest as the Delta will be potentially moving from 1.00 toward 0 or vice versa as the underlying crosses a strike. In these cases, the Gamma can be extremely high as the Delta changes rapidly with the underlying at the strike and expiration approaching.

Deep-in-the-money or far-out-of-the-money options have lower Gamma than at- the-money options. The deep-in-the-money options already have a high positive or negative Delta. If the options become deeper in-the-money, the Delta will move toward 1.00 (or -1.00 for puts) and the Gamma will decrease because the Delta cannot move past 1.00. If the stock were to move toward the strike of the deep-in-the-money option, the Gamma will increase and the Delta moves lower approximately by the amount of the current Gamma.

For example, let’s say XYZ is trading at $30. A 25 strike call is trading for $5.80 and has a Delta around .85 and a Gamma of .03. If XYZ were to drop to $29, the investor might expect the option premium to drop to $4.95 (as projected by Delta). With stock at $29, the Delta of the option will be decreasing by approximately the amount of the Gamma, so the new Delta at an XYZ price of $29 might be .82 (here we subtract the Gamma from the old Delta as the stock price declined by $1). With the stock moving down toward the long strike, Gamma increases and impacts Delta. Should the stock decline to $25, we’d estimate Delta to be around .50. If the Gamma stayed around .03, the option would still have a .70 Delta with the stock at $25. However, since Gamma typically increases as options become closer to at-the-money, the new Gamma of this contract may be around .09.

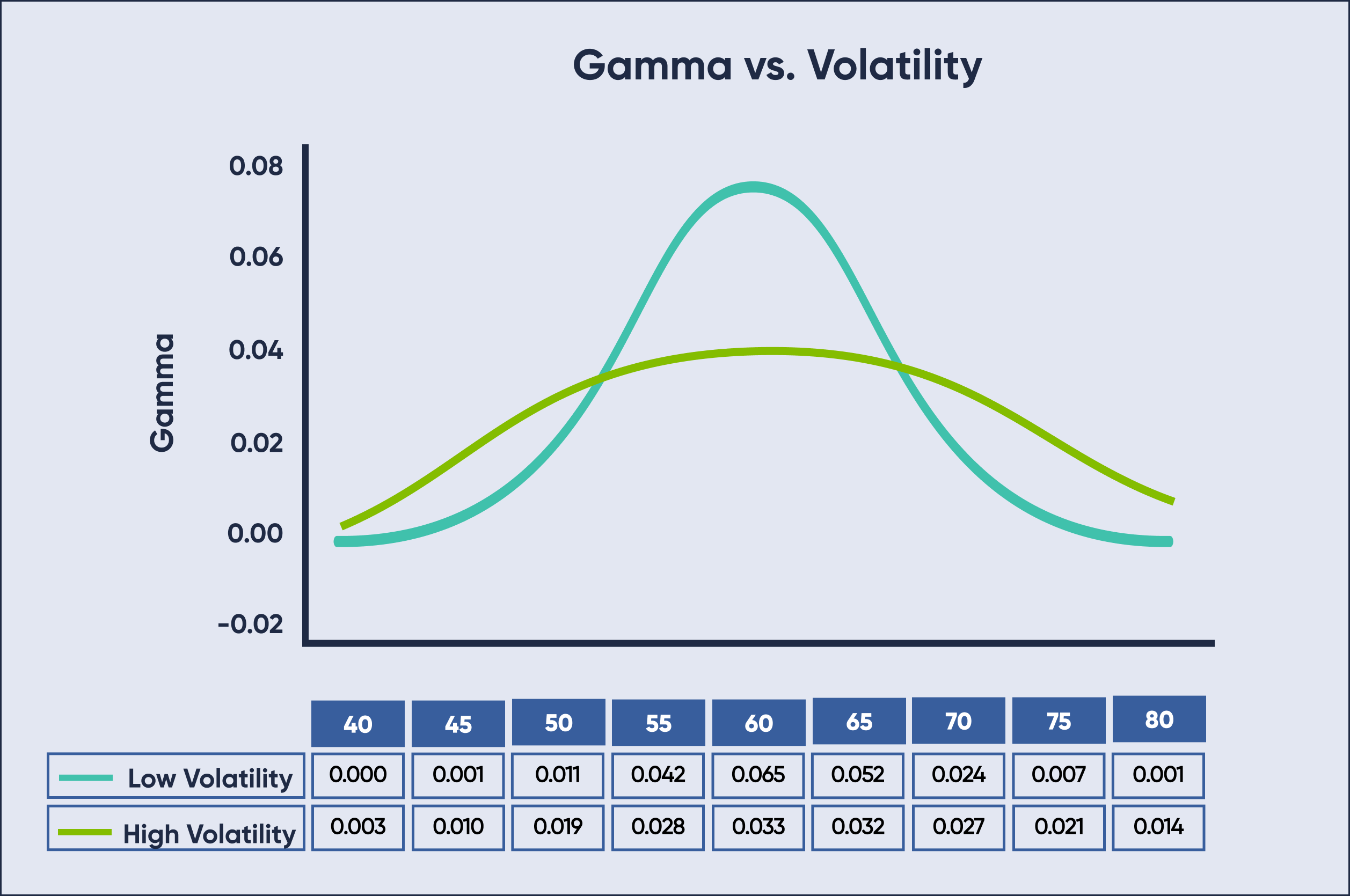
Gamma is highest when the Delta is in the .40-.60 range, or typically when an option is at-the-money. Deeper-in-the-money or farther-out-of-the-money options have lower Gamma as their Deltas will not change as quickly with movement in the underlying. As Deltas approach 0 or 1.00 (or 0 or -1.00 for puts), Gamma is usually at its lowest point.

Implied volatility changes will also have an effect on Gamma. As implied volatility decreases, Gamma of at-the-money calls and puts increases. When implied volatility goes higher, the Gamma of both in-the-money and out-of-the-money calls and puts will be decreasing. This occurs because low implied volatility options will have a more dramatic change in Delta when the underlying moves. A high implied volatility underlying product will see less of a Delta change with movement as the possibility of more movement is foreseen.